How To Draw Centroid
How To Draw Centroid - A triangle a b c has the sides a b = 6, a c = 4 and b c = 7. 🙋 in a triangle, the centroid is the point at which all three medians intersect. Web to find the centroid of a triangle algebraically, we need to draw three medians one from each vertex of the triangle to the midpoint of their opposite sides. Construct the perpendicular bisectors of two sides ac and bc to find the mid points d and e of ac and bc respectively. There are a few different ways to find the centroid of a triangle. The centroid has an interesting property besides being a balancing point for the triangle. I will draw another altitude. Web how to construct the centroid of a triangle. Draw triangle abc using the given measurements. Their intersection is the centroid. It is referred to as the center of mass or balance point of the triangle. Web to locate the centroid, draw each of the three medians (which connect the vertices of the triangle to the midpoints of the opposite sides). Construct the perpendicular bisectors of two sides ac and bc to find the mid points d and e of ac. Where all three lines intersect is the centroid , which is also the center of mass: Web to find the centroid of a triangle algebraically, we need to draw three medians one from each vertex of the triangle to the midpoint of their opposite sides. Choose a side of the triangle. There are a few different ways to find the. The lines will intersect at the centroid. 24k views 5 years ago geometry for sixth class onwards. Construct a 90° angle (right angle) sum of n angles. Web the centroid is the intersection of the medians, which divide the triangle into six congruent triangles. Centroid of semicircle using integration formulas. Now let's draw the altitudes of this triangle. Now, the point g is the centroid of the given triangle abc. 🙋 in a triangle, the centroid is the point at which all three medians intersect. These line segments are the medians. Their intersection is the centroid. Therefore, the orthocenter and the centroid coincide only when the triangle has three equal sides and angles. Constructing 75° 105° 120° 135° 150° angles and more. Centroid of a tee section. What is the easiest way to find the centroid of a triangle? Set the compass to a length greater than half the length of ab. Web how to construct the centroid of a triangle. A triangle a b c has the sides a b = 6, a c = 4 and b c = 7. The coordinates of the centroid are g ( x, y) = ( x 1 + x 2 + x 3 3, y 1 + y 2 + y 3 3) g ( x, y) = ( 3 + 2 + 0 3, 2 − 3 + 0 3) = ( 5 3, − 1 3) in the triangle given below if g is the centroid and ag =. The lines will intersect at the centroid. Construct the centroid of the triangle. How do you find the centroid of a triangle?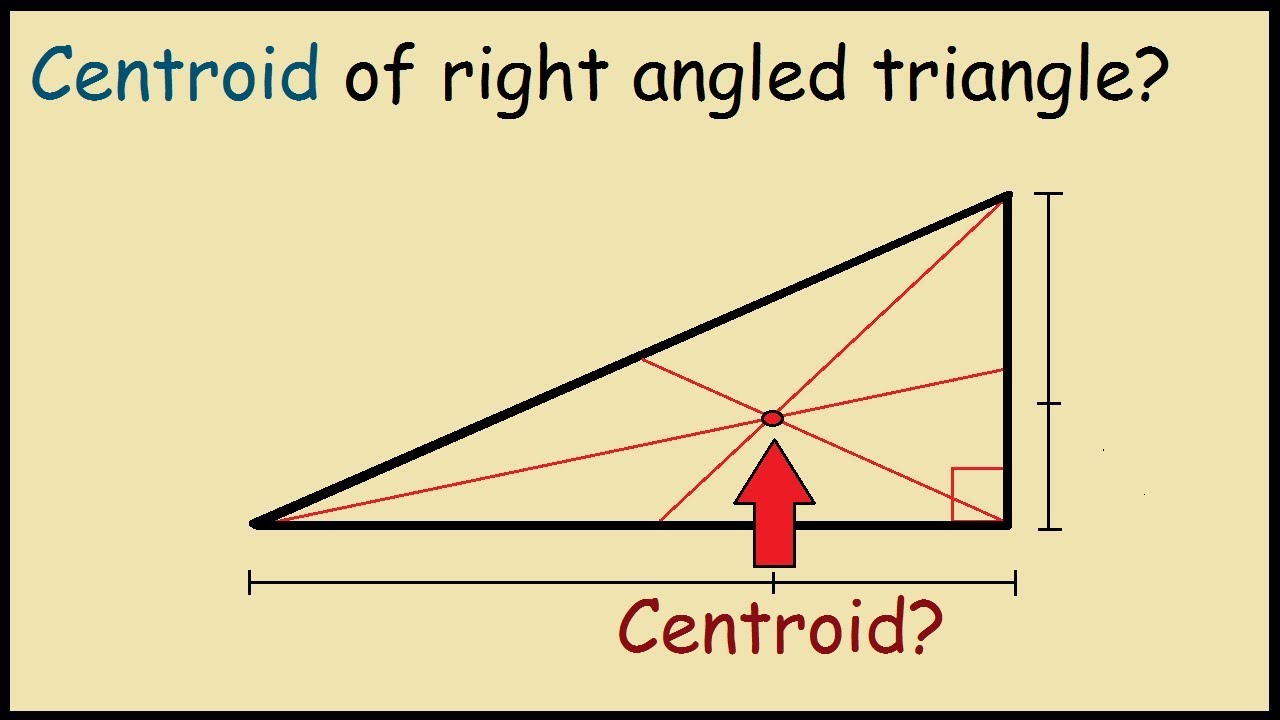
Centroid Of Triangle

Centroid of a Triangle Definition, Differences, Properties, Examples

Centroid of a Triangle Definition, Differences, Properties, Examples
So Let's Draw The Altitude.
In This Video I Show You How To Do Construction 21 From The Leaving Cert Higher Level Course Which Is To.
Web The Centroid Is Also Known As The Geometric Center Of The Object.
Centroid Of A Right Triangle Using Integration Formulas.
Related Post: